The machining method of the eccentric modification gear generated by the modification method is the same as that of the elliptical and non-circular gears. The essence of the machining is that the straight line of the rack cutter pitch is tangent to the curve of the eccentric gear pitch and it is pure rolling. A modification MX must be added in the vertical direction of the rack cutter to envelope the involute profile of the eccentric modification gear.
The number of teeth of the gear is designed to be odd. In the initial position, one end of the long shaft of the active eccentric gear is a tooth, and the conjugate eccentric displacement gear corresponds to a tooth slot. During the computer simulation processing, the wheel I rotates clockwise around the axis, and the wheel II rotates counterclockwise around the axis. The rack cutter moves downward at the same time, and the distance of downward translation is the arc length corresponding to the gear rotation angle; at the same time, it moves horizontally and rotates at a certain angle to ensure that the straight line of the rack section is tangent to the two curves.
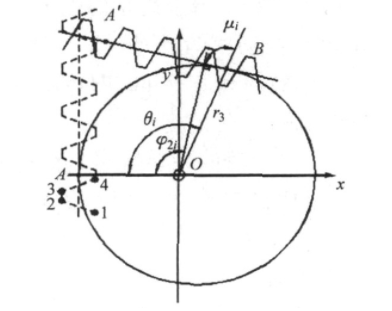
Suppose that the gear blank of the eccentric modification gear is fixed, and the gear rack cutter pitch rolls around the gear blank curve in a straight line. Fig. 1 shows the position relationship between the gear rack cutter and the eccentric modification gear.
Polar equation of pitch curve of eccentric gear II
r2=R2-e2sin2φ2+ecosφ2
Then the polar equation of the pitch curve of the eccentric modified gear is
r3=R2-e2sin2φ2+ecosφ2+mx (7)
When it is set at the starting position, the pitch line of the rack cutter is tangent to the pitch curve of the eccentric gear blank at point A. when the pitch line of the rack cutter rolls purely along the pitch curve to the polar angle φ 2I, it is tangent to the pitch curve of the gear blank at point B. the angle μ I between the pitch line and the diametral vector ob is [12]
μi=arctanr3 (φ2i) (dr3dφ2) φ2i (8)
When point a moves to point a ‘, the arc length s between AB is the same as the distance between point a ′ B, that is
S=lA′B=∫0φ2ir32+ (dr3/dφ2) 2dφ2 (9)
The angle θ I of the rack is
θi=φ2i+μi-π2 (10)
The pitch curve of the workpiece gear II can be enveloped by rotating the rack once according to the formula (8) – (10).
On the basis of the straight line envelope of the gear pitch curve of the workpiece, the rack profile envelope the involute profile of the gear. The tool rack is composed of several teeth, each of which can be represented by four vertices (Fig. 4). The first tooth is composed of four vertices, i.e. 1, 2, 3 and 4. The other teeth are obtained from the first tooth through coordinate translation. When the arc length s corresponding to the angular displacement φ 2 of the blank is greater than one pitch P or the integral times of the pitch, the coordinate y vector of the rack cutter moves forward the integral times of the pitch P to ensure that there are enough rack teeth to envelop the gear every time. In the rack coordinate system, the mathematical model of tool rack is expressed in matrix form as
x0=1.25m[1 -1 -1 1]
y0=[-3πm4-lm -3πm4+lm -πm4-lm -πm4+lm]+nπm (11)
Where LM = 1.25mtan α
Where n — number of teeth of rack
If the rack is turned to the tangent direction of the gear blank pitch curve, the mathematical model is
{x1=x0cos (μ-π/2) - (y0-S+nπm) sin (μ-π/2) y1=x0sin (μ-π/2) - (y0-S+nπm) cos (μ-π/2) (12)
Put the rack to the position tangent to the gear blank and make pure rolling, and then offset MX for displacement, then the mathematical model is
{x3= (x1+r2+mx) cosφ2-y1sinφ2y3= (x1+r2+mx) sinφ2+y1cosφ2 (13)
With the constant change of φ 2, the rack rotates once to envelope the profile of eccentric displacement gear, as shown in Fig. 2.

The involute tooth profile is connected by the intersection points of the enveloping lines. The top curve of the eccentric modification gear is a normal equidistant line with the distance from the pitch curve of MH * a, and the transition curve of the tooth root is formed by enveloping the vertex of the top line of the rack. Thus, the tooth root transition curve of all the teeth of the gear, the involute tooth profile and the characteristic points of the tooth profile of the top curve are successively connected into a complete tooth profile.
