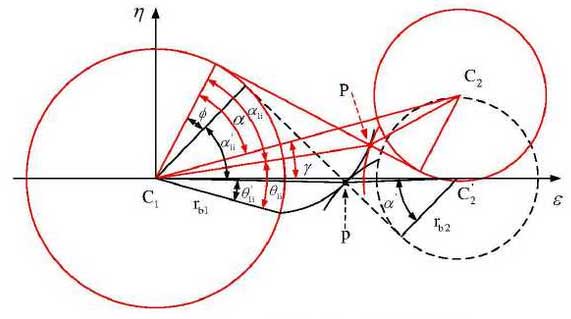
During gear transmission, changes in center distance cause changes in the direction of the engagement line, resulting in the actual engagement point P deviating from the engagement point P in the figure, as shown in the figure.In the figure C1 and C2 are respectively the position of the center of mass of the two gears after the center distance changes. In the figure, the theoretical center position of C2 gear 2 relative to gear 1 is satisfied with the angle_:

In the equation, alphaPli is the pressure angle of the actual engagement point P; alphapli is the pressure angle of the engagement point p, which is calculated from the formula; theta Pli and theta PLI are the involute spread angles of the engagement point P and the engagement point p, respectively.
By substituting the function theta=tanalpha-alpha of the involute into the formula, we can get:
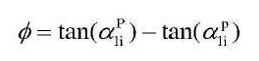
In the figure, the angle phi satisfies both:

Where sign is a sign function, alpha is the theoretical engagement angle, alpha is the dynamic engagement angle, calculated by formula, and position angle is calculated by formula.
The pressure angle of the actual engagement point at any time can be obtained as follows:

