In order to intuitively analyze the generation principle of right spiral bevel gear convex tooth surface, the method of establishing concave tooth surface generation model is used, and the 3D generation model of right spiral bevel gear convex tooth surface is established by using the wire frame and surface design module, assembly design module and DMU kinematics module of CATIA software, as shown in the figure. (Q) The plane is tangent to the base cone, and the center of the (q) plane coincides with the top of the base cone at the o ‘point. When the base cone is fixed and the (q) plane rolls purely on the base cone at the rotation speed of ω ‘, the generating line of the tooth surface – a’ B ‘will roll purely on the base cone with the (q) plane, thus generating the convex tooth surface of the right-hand spiral bevel gear. The whole generation process of the convex tooth surface is that the generation line of the tooth surface starts from the a ‘of the small end to the B’ of the large end and wraps on the base cone, and then expands from the base cone to the face cone to generate the convex tooth surface model.
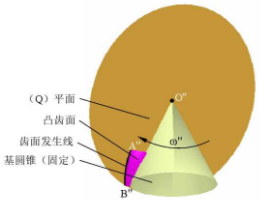
By comparing the generation process of the concave tooth surface and the convex tooth surface of the right-hand spiral bevel gear, there are the following differences: ① the concave tooth surface is wound on the base cone from the big end to the small end of the generation line of the tooth surface; while the convex tooth surface is just the opposite, winding from the small end to the big end; ② when the base cone is fixed, the (q) plane in the generation process is in the base circle The direction of pure rolling on the cone is opposite. The same points are as follows: ① the convex and concave tooth surfaces are generated from the generating line of the tooth surface from the base cone to the face cone; ② the center of the (q) plane coincides with the top of the base cone, and the (q) plane is always tangent to the base cone, and pure rolling motion is carried out to generate the tooth surface of the spiral bevel gear.
In the same analysis method as concave tooth surface, the spatial rotation motion of (q) plane on the base cone in the process of generating convex tooth surface is decomposed into two plane rotation motions, as shown in the figure. (Q) The plane is tangent to the base cone, and the center of the (q) plane coincides with the top of the base cone at the o ” point. When the (q) plane and the base cone rotate at the angular velocity ω ‘and ω 1 around their own geometric axis respectively at the angular velocity ω’ and ω 1 ‘, the (q) tooth generating line of the plane arc segment a’ B ” will roll purely on the base cone, and the arc segment The spatial motion path of a ‘B’ relative to the base cone is the convex tooth surface of the right spiral bevel gear. Based on the motion analysis of the generation process of the concave tooth surface, it can be seen that in order to ensure the pure rolling relationship between the (q) plane and the base cone.
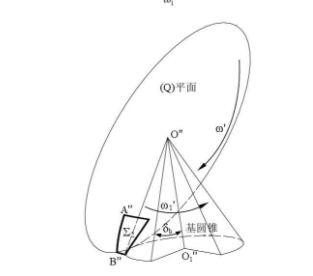
The rotation motion of the base cone and the (q) plane needed in the generation process of the convex and concave tooth surfaces of the right-hand spiral bevel gear is compared and analyzed, and the following conclusions are drawn: ① the rotation speed direction of the base cone and the rotation speed direction of the (q) plane are opposite, and the speed of both are the sine value of the base cone angle; ② the rotation speed of the base cone is the same as that of the (q) plane The direction of rotation of the (q) plane and the base cone is exactly opposite to the generated convex tooth surface, that is, the pure rolling direction of the generated concave tooth surface is opposite to the generated convex tooth surface.
