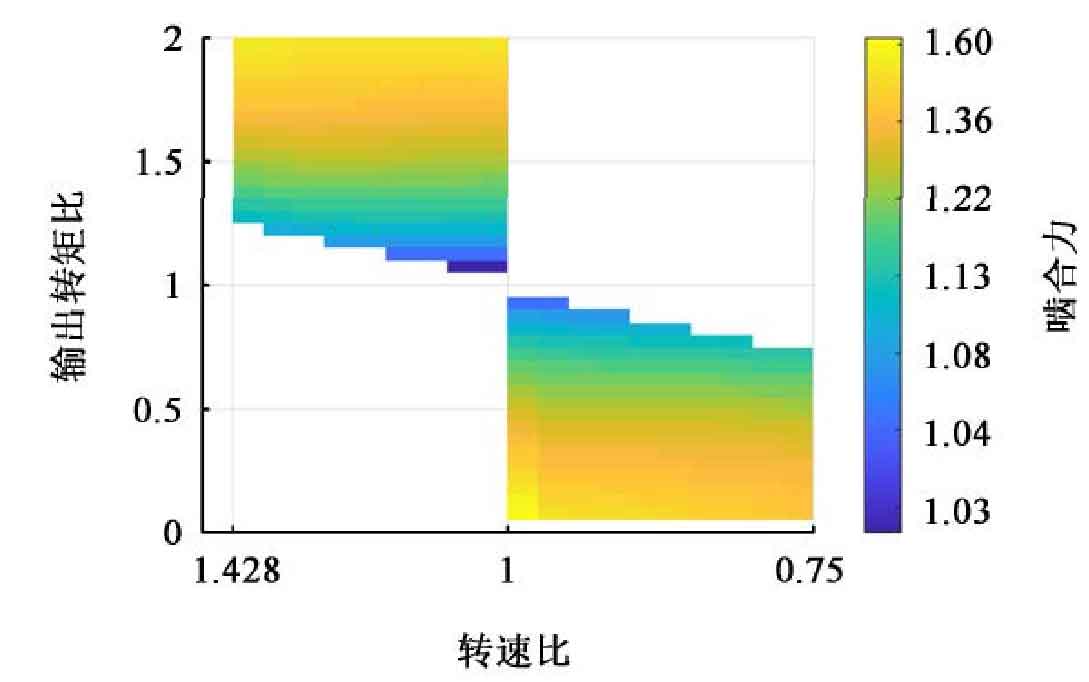
The maximum meshing force reflects the maximum load of planetary gear. The dynamic load coefficient is the ratio of the maximum value of the dynamic meshing force in one system cycle to the static transmission value of the meshing force, which can reflect the vibration of the gear pair. The larger the amplitude of dynamic load coefficient is, the greater the vibration in the direction of meshing line is.
As shown in the figure, the maximum value of the meshing force is the combination of the assumed theoretical meshing force in P10 and P20 rows according to the frequency ratio. As shown in the formula, it is called the actual meshing force. The dynamic load coefficient of the meshing force can be obtained by dividing the synthetic actual meshing force by the assumed theoretical meshing force
Where GP10 and GP20 are the dynamic load coefficients of two rows of planetary gears respectively.
Figure 2 shows the dynamic load coefficient of engagement force. The color axis in Figure 2 shows the dynamic load coefficient. The dynamic load coefficient of P10 increases with the decrease of output torque ratio and speed ratio. The maximum value of dynamic load coefficient is the position with the minimum speed ratio and load ratio, and the value is 13.18. This condition is small radius steering, P10 row is the low speed side, and P10 row resistance is 1 / 10 of P20 row resistance. The distribution of dynamic load coefficient of P20 is opposite to that of P10, which decreases with the decrease of output torque ratio and speed ratio. The maximum value is 2.58, which occurs when turning with small radius, P20 is low speed, and the resistance of P20 is 1 / 2 of that of P10.
When P10 is on the high speed side (the speed ratio is greater than 1), the dynamic load coefficient of P20 is larger. When P20 is on the high speed side (the speed ratio is less than 1), the dynamic load coefficient of P10 is larger. The maximum dynamic load coefficient of the two rows is close to 5 times, which is the same as the resistance difference. The relationship between dynamic load coefficient and speed ratio, output torque ratio can not be expressed by the difference between the two factors and 1. When the output torque ratio is less than 1, the dynamic load coefficient is a multiple of its reciprocal.
According to the joint analysis of Fig. 1 and Fig. 2, when P10 is a high-speed row, the meshing forces of the two rows are similar, but the dynamic load coefficient of P20 row is greater than that of P10 row. At this time, the vibration response of P20 row may be greater than that of P10 row, and the turning radius is small. When P10 row has larger resistance, the vibration response is larger; When P20 is high-speed, P10 has a large dynamic load coefficient. When the torque ratio is less than 0.2, the dynamic load coefficient is greater than 5, and the meshing force is greater than 1.36, which may cause large vibration.
In conclusion, the engaging forces of the two rows are similar in the steering process, but the dynamic load coefficient of the low-speed side is larger than that of the high-speed side. The smaller the steering radius is, the larger the difference of output torque is, and the larger the dynamic load coefficient is. The vehicle should try to avoid working in the working area with smaller steering radius and larger difference of output torque.