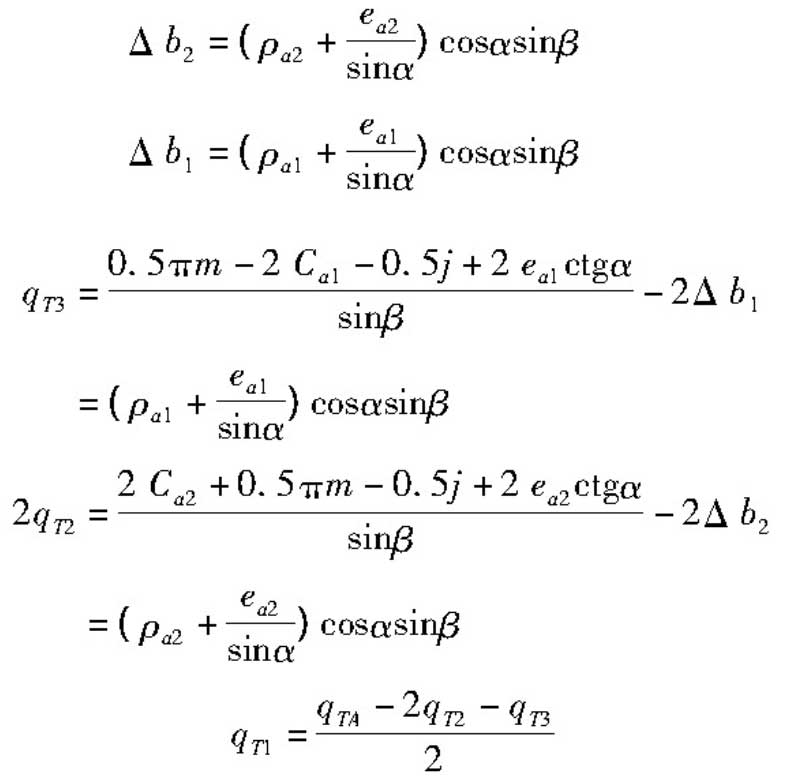The working tooth profile of the hexagon-arc helical gear is composed of six segments of circular arcs. It adopts the step-by-step tooth type and tangentially displaces the two adjacent tooth profiles in the direction of tooth height. The transition between the two adjacent tooth profiles is made by connecting circular arcs. The basic tooth profile of the hexagon-arc helical gear is shown in Figure 1.The three circular arc profiles above the pitch line are convex profiles, the three circular arc profiles below the pitch line are concave profiles, and the two adjacent tooth profiles are connected by transitional circular arcs.
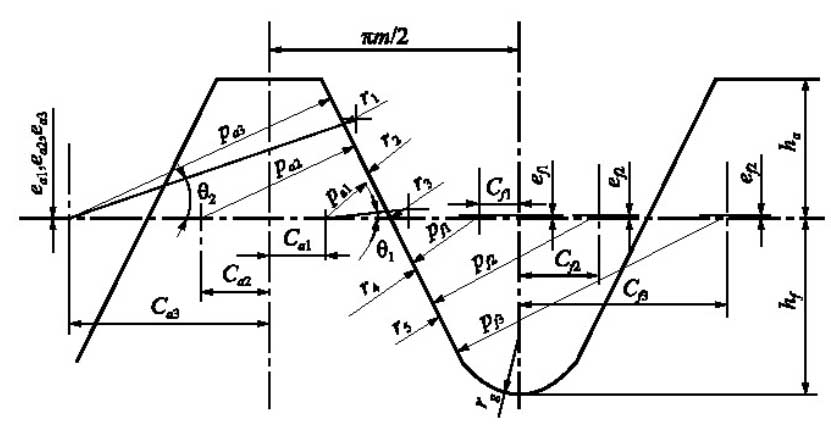
Parameters in the drawing: Profile arc radius of convex teeth 1, Profile arc radius of convex teeth 2, Profile arc radius of convex teeth 2, Profile arc radius of convex teeth 3, Profile arc radius of concave teeth 1, Profile arc radius of concave teeth 2, Profile arc radius of concave teeth 3, Profile arc radius of concave teeth 3, Profile center offset ea1, ea2, ea3, Ca2, Ca3, Profile center offset of concave teeth ef1, ef2, Cf2, Cf3, Process angle of convex teeth theta 1, Theta 2;Connect the arc radius r1, r2, r3; root arc radius rg.
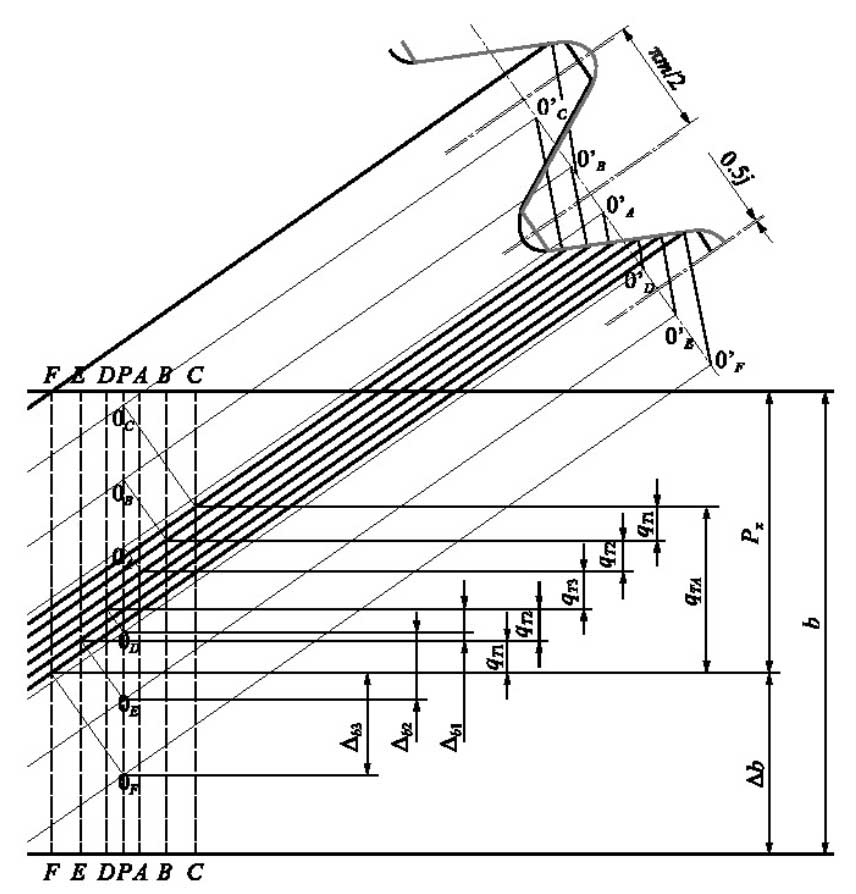
To calculate the engagement characteristics of six-arc helical gears, it is first necessary to calculate the axial distance between the six engagement points on the same gear tooth. Figure 2 shows the tooth profile of the basic rack of the six-arc helical gears in the normal plane and the plane diagram of the cylindrical expansion of the gear pitch. PP is the pitch of the gear, and three engagement lines of the convex teeth AA, BB, CC and concave teeth DD, EE and FF are distributed on both sides of the pitch line respectively.The cylindrical helix at which the gear engagement point locates develops and becomes a straight line with helical angle beta included with the engagement line. The intersection point of the contact line and the engagement line on the pitch plane is the engagement point of the gear.
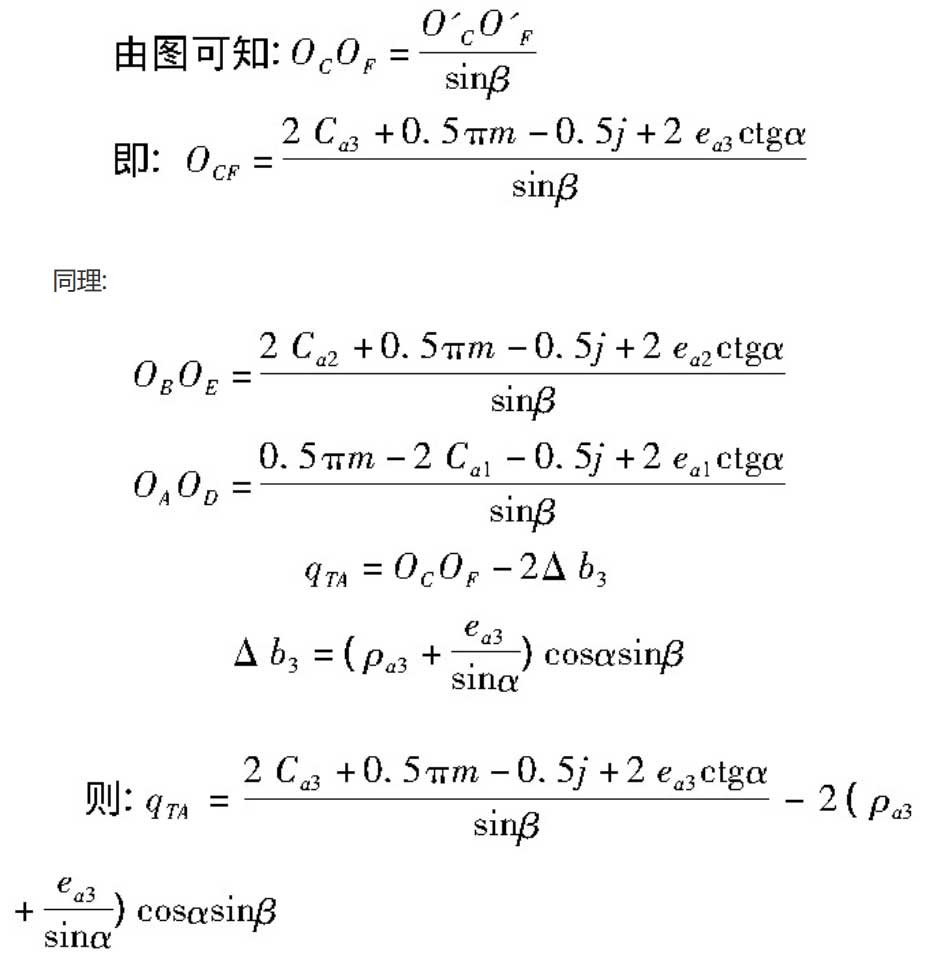
The maximum axial distance J2CJ2F between the six simultaneous contact points on the same tooth is qTA, the two axial distances between the engagement points of the three convex tooth surfaces are J2CJ2B, and the J2BJ2A are qT1 and qT2 respectively.The axial distance between the engagement point J2AJ2D of concave and convex two teeth is qT3. The axial distance between the adjacent two teeth contact point J1FJ2C is recorded as q’TA where q’TA=Px-qTA.