The rationality of the proposed method is verified by the nonlinear vibration reliability analysis of a pair of standard spur gears.
Each random parameter and its distribution type in the gear transmission equation, the mean value of each data comes from the deterministic calculation results, and the standard deviation is 1 / 10 of the mean value, that is, the coefficient of variation of 0.1 is adopted.
SD, SV and SA are the safety thresholds of vibration displacement, vibration velocity and vibration acceleration respectively.
The safety threshold of each nonlinear vibration response is determined as follows.
1) According to the random parameters of the model, sampling and several times of simulation, such as 50 times of simulation, the variation range of each nonlinear vibration response is obtained.
2) According to the simulation, the variation range of the nonlinear vibration response is obtained. The upper and lower bounds of the safety threshold are 95% of the upper and lower bounds respectively.

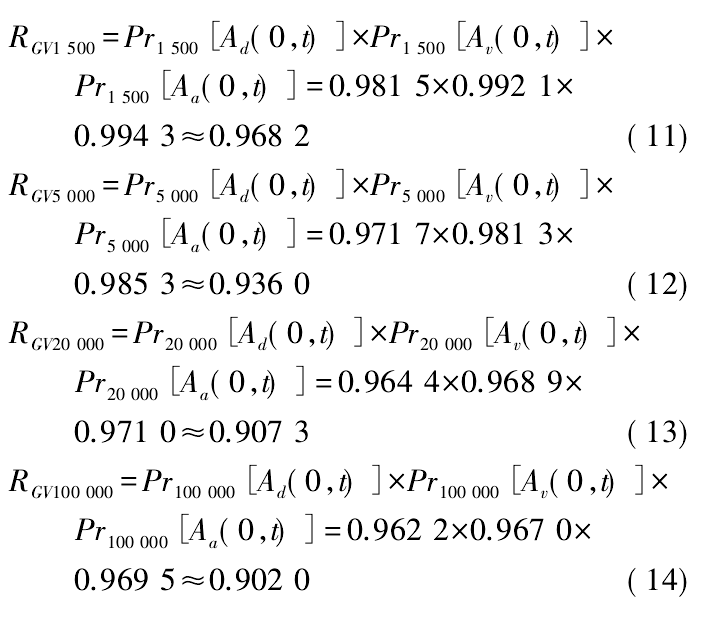
Each nonlinear vibration response of gear transmission is simulated and solved according to the solution flow of the simulation method. The simulation step size Δ t is set as 0.05 s, and 1 500, 5 000, 20 000 and 100 000 times of simulation analysis are carried out respectively. The variation law of nonlinear vibration response reliability with simulation times is shown in Fig. 2; The calculation results of gear nonlinear vibration reliability under different simulation times are shown in the formula.
1) Since the nonlinear vibration response of gear is usually chaotic, it is difficult to describe it by steady-state stochastic process. Therefore, it is recommended to use the multi pass Monte Carlo simulation method proposed in this paper in practical engineering.
2) It can be seen from the calculation results in the formula that,
The simulation results tend to converge after 20 000 cycles (about 0.9073). Because the reliability of gear nonlinear vibration is calculated systematically, the result is safe.
