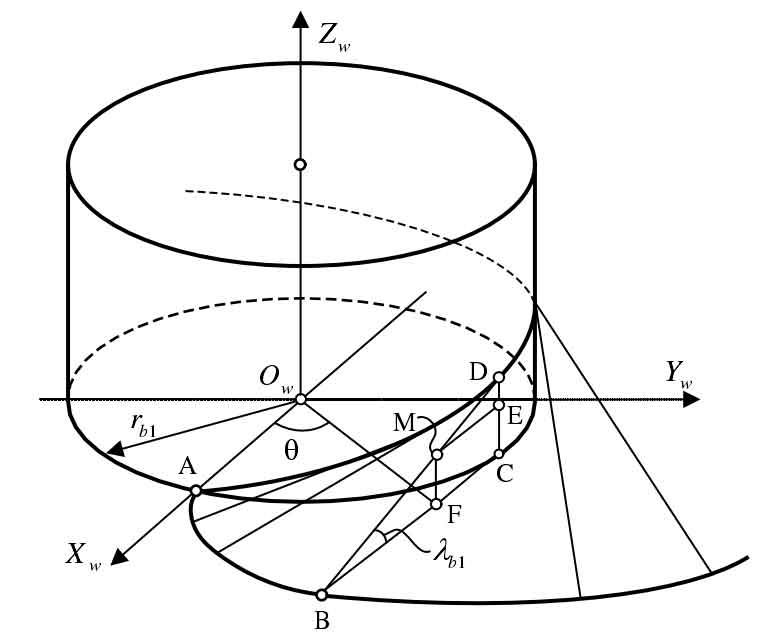
Based on the selection of basic worm, it is determined that the gear hob selected in this subject is involute worm hob. In order to simplify the calculation, the coordinate system in line with this study is established. Gear hob coordinate system: SW, (ow: XW, YW, ZW), take the gear hob axis as the ZW axis, take the position of the starting point a of the end involute as the positive direction of the XW axis, then the intersection of the end section of point a and the ZW axis is set as the origin ow of the gear hob coordinate system. Finally, the positive direction of the YW axis is determined by the right-hand rule of the Cartesian coordinate system. The coordinate system of involute helicoid model of gear hob is shown in the figure.
From the knowledge of spatial geometry, a spatial surface can be determined by two variables. Therefore, the involute helical surface of gear hob is also determined by two variables. One of the variables is the polar diameter ρ, Expressed as the length of the line segment OWF, and point F is the projection of any point m on the involute helical surface of the gear hob on the xwwyw plane.
Another variable is polar angle θ , Is the included angle between the forward direction of XW axis and OWF of gear hob coordinate system. AB is the intersection of involute helicoid and xwwyw plane, and ab is an involute in the section of gear hob end. At any point m on the involute helicoid, make the tangent plane BCD of the base cylinder through the point m, the tangent between the tangent plane BCD and the base cylinder is DC, and the intersection of the tangent plane BCD and xwwywyw plane is BC. According to the properties of the tangent plane, DC is parallel to ZW axis. Point E is the vertical foot of point m on DC.