This paper analyzes the tooth surface equation, conjugate tooth surface equation and tooth surface contact line equation of involute arc tooth cylindrical gear. Whether these expressions are correct needs to be verified by some methods. The method to describe the meshing characteristics of gear tooth surface is to express it graphically, that is to say, its mathematical expression is expressed in the form of graph, so that the meshing characteristics of gear tooth surface can be analyzed intuitively. The method is to discretize the continuous curved surface or curve by computer programming, take several discrete points, draw the spatial position of the points, and connect the similar points with straight lines. As long as the discrete distance is small enough, the meshing characteristics of the gear tooth surface can be well represented.
The single tooth surface figure discretized according to the formula is shown in Figure 1. The gear pair is a non displacement gear with modulus M = 4mm, number of teeth Z1 = 20, tooth width b = 40mm, and tooth line arc radius RT = 30mm.
According to the gear tooth surface equation, conjugate tooth surface equation and meshing line equation, the meshing line graph of the tooth profile discretized is shown in Figure 2. The module of the gear pair is m = 4mm, the number of teeth is Z1 = 20, Z2 = 40, and the arc radius of the tooth line is RT = 30mm.
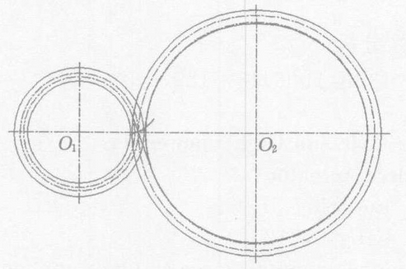
Enlarge the meshing area of Fig. 2 to Fig. 3, and clearly observe the tooth profile 1, conjugate tooth profile 2 and meshing line 3 of the pinion in Fig. 3. The tooth profile 1 and the tooth profile 2 are tangent, and the tangent point is k point, which is also the intersection of the two tooth profiles and the meshing line 3. The tooth profile meshing line 3 can be regarded as a straight line tangent to the base circle of two gears. Figure 3 depicts the instantaneous state of pinion angle φ 1 = 0 ° and pinion profile engagement angle α = 20 °.

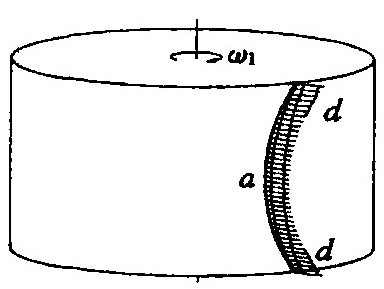
According to the tooth surface equation and contact line equation of the gear, the discrete tooth surface contact line figure is shown in Figure 1. The module of the gear is 4 m m, the number of teeth is Z1 = 20, the radius of arc of the tooth line is RT = 30 mm, and the number of teeth of the gear engaged with it is Z2 = 40.
Figure 1 shows four contact lines of tooth surface engagement, which are respectively contact lines in different states. Contact line a is the contact line when it just enters the tooth surface engagement, and contact line D is the contact line when it is about to exit the tooth surface engagement.
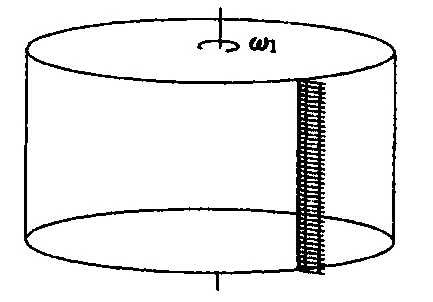
Figure 4 shows the contact line between the single tooth surface and the tooth surface of the spur gear. The contact line is a straight line, and the length of the contact line is less than the length of the contact line of the arc tooth surface. In addition, the arc gear pair has the axial engagement coincidence degree, which is greater than that of the straight gear pair with the same module and number of teeth. In the case of the same tooth width, the smaller the arc radius of the tooth line, the greater the engagement coincidence degree of the arc gear pair.
This paper analyzes the tooth surface equation, conjugate tooth surface equation and tooth surface contact line equation of involute arc tooth cylindrical gear, and expresses the mathematical expression in the form of graph, so that the meshing characteristics of gear tooth surface can be analyzed intuitively. It lays a foundation for the study of the strength and machining method of the spiral gear.
It can be seen from Fig. 1 that the contact line of the tooth surface of the arc tooth cylindrical gear is in the shape of spatial curve, the contact line of the tooth surface is long, and symmetrically distributed with respect to the middle section of the gear. Therefore, the contact strength and the bearing capacity of the tooth surface of the gear are better than that of the straight or helical cylindrical gear. From Fig. 3, it can be found that if the diameter of gear pitch circle is constant, properly increasing the diameter of tooth top circle and reducing the diameter of tooth root circle can increase the actual meshing line of gear pair and increase the meshing coincidence degree of gear pair. The involute arc tooth cylindrical gear is a new type of gear, which has better transmission characteristics, and will replace the application of straight or helical cylindrical gear in many occasions.
